Introduction to Probability questions and answers pdf
Posted on 18th Feb 2019 20:02:46 in Questions and Answers
Probability Questions with Solutions
Tutorial on finding the probability of an event. In what follows, S is the sample space of the experiment in question and E is the event of interest. n(S) is the number of elements in the sample space S and n(E) is the number of elements in the event E.
in these lessons, we will learn how to solve some probability word problems.
• Probability and Sample Space
• Probability and Frequency Table
• Probability and Area
• Probability of Simple Events
• Probability and Permutations
• Probability and Combinations
• Probability of Independent Events
We will now look at some examples of probability problems.
Example:
At a car park there are 100 vehicles, 60 of which are cars, 30 are vans and the remainder are lorries. If every vehicle is equally likely to leave, find the probability of:
a) van leaving first.
b) lorry leaving first.
c) car leaving second if either a lorry or van had left first.
Solution:
a) Let S be the sample space and A be the event of a van leaving first.
n(S) = 100
n(A) = 30
Probability of a van leaving first:
b) Let B be the event of a lorry leaving first.
n(B) = 100 – 60 – 30 = 10
Probability of a lorry leaving first:
c) If either a lorry or van had left first, then there would be 99 vehicles remaining, 60 of which are cars. Let T be the sample space and C be the event of a car leaving.
n(T) = 99
n(C) = 60
Probability of a car leaving after a lorry or van has left:
![]()
Example:
A survey was taken on 30 classes at a school to find the total number of left-handed students in each class. The table below shows the results:
|
No. of left-handed students |
0 |
1 |
2 |
3 |
4 |
5 |
|
Frequency (no. of classes) |
1 |
2 |
5 |
12 |
8 |
2 |
A class was selected at random.
a) Find the probability that the class has 2 left-handed students.
b) What is the probability that the class has at least 3 left-handed students?
c) Given that the total number of students in the 30 classes is 960, find the probability that a student randomly chosen from these 30 classes is left-handed.
Solution:
a) Let S be the sample space and A be the event of a class having 2 left-handed students.
n(S) = 30
n(A) = 5 ![]()
b) Let B be the event of a class having at least 3 left-handed students.
n(B) = 12 + 8 + 2 = 22 ![]()
c) First find the total number of left-handed students:
|
No. of left-handed students, x |
0 |
1 |
2 |
3 |
4 |
5 |
| Frequency, f (no. of classes) |
1 |
2 |
5 |
12 |
8 |
2 |
|
fx |
0 |
2 |
10 |
36 |
32 |
10 |
Total no. of left-handed students = 2 + 10 + 36 + 32 + 10 = 90
Here, the sample space is the total number of students in the 30 classes, which was given as 960.
Let T be the sample space and C be the event that a student is left-handed.
n(T) = 960
n(C) = 90 ![]()
Probability and Area
Example:
ABCD is a square. M is the midpoint of BC and N is the midpoint of CD. A point is selected at random in the square. Calculate the probability that it lies in the triangle MCN.
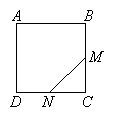
Solution:
Let 2x be the length of the square.
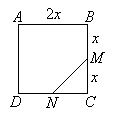
Area of square = 2x × 2x = 4x2
Area of triangle MCN is ![]()
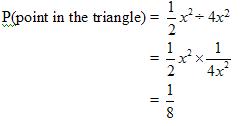
This video shows some examples of probability based on area